CLASS-4
CIRCLE
CIRCLE -
A circle is a closed two-dimensional geometric shape consisting of all the points that are equidistant from a fixed point called the center. The distance between the center and any point on the circle is called the radius. The circumference of a circle is the distance around the edge of the circle, and it is equal to 2π times the radius. Circles have many important properties, including symmetry and the fact that any diameter (a line segment passing through the center) divides the circle into two equal halves.
A circle is a geometrical shape consisting of all points in a plane that are equidistant from a given point called the center of the circle. A circle is a closed figure and has no corners or edges. The distance from the center to any point on the circle is called the radius of the circle. The distance across the circle passing through the center is called the diameter. The circumference of a circle is the distance around the circle, which is equal to 2π times the radius, where π (pi) is a mathematical constant approximately equal to 3.14159. Circles have many applications in mathematics, science, and engineering, and are commonly used to represent various things, such as the orbits of planets or the shape of wheels.
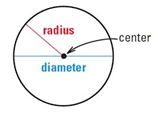
A circle is
a simple closed curve with a center.
~ Center is the fixed point of the circle in the given plane from which every point on the curve is equidistant.
~ The length of the boundary of a circle is known as its circumference.
~ The distance between the center and any point on the circle is called the radius of the circle.
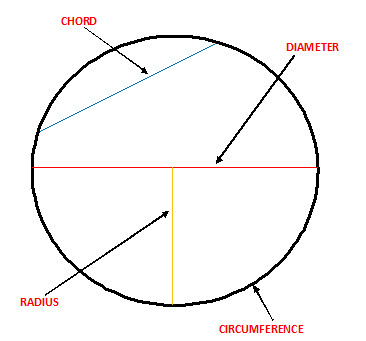
~ All the radii of the same circle are equal in length.
~ The straight line which passes through the center of the circle with its end points lying on its circumference is known as the diameter.
~ All the diameters of the same circle are equal in length.
~ Half of the circle is called semi-circle.
~ Any line segment joining two points on the circle is called a chord. Diameter is the longest chord of the circle.
~ RELATION BETWEEN DIAMETER AND RADIUS ~
Diameter = 2 x Radius
𝑫𝒊𝒂𝒎𝒆𝒕𝒆𝒓
Radius = --------------
𝟐
Example.1) If the diameter of a circle is 30 cm then find the value of radius
Ans.) The diameter of circle is 30 cm given.
As per the formulae radius = diameter/2
so, Radius = 30/2 = 15 cm
So, the radius of the circle is 15 cm (Ans.)
Example.2) If the radius of a circle is 10 cm, then find the diameter of the circle.
Ans.) The radius of the circle 10 cm is given.
as per the formulae diameter = radius X 2
so, diameter = 10 X 2 = 20 cm
So, the diameter of the said circle is 20 cm (Ans.)