CLASS-4
PERIMETER OF A RECTANGLE
PERIMETER OF A RECTANGLE -
A rectangle is a two-dimensional shape with four sides, where each pair of opposite sides are parallel and congruent. The parameters of a rectangle refer to the characteristics that describe its size and shape.
The main parameters of a rectangle are:
- Length: The length is the distance between the two parallel and congruent sides of the rectangle. It is usually denoted by "l" or "L".
- Width: The width is the distance between the other two parallel and congruent sides of the rectangle. It is usually denoted by "w" or "W".
- Perimeter: The perimeter of a rectangle is the total distance around the outside of the rectangle. It is calculated by adding the lengths of all four sides of the rectangle. The formula for the perimeter of a rectangle is: P = 2(l + w).
- Area: The area of a rectangle is the amount of space inside the rectangle. It is calculated by multiplying the length and width of the rectangle. The formula for the area of a rectangle is: A = l x w.
The perimeter of a rectangle is the total length of its four sides.
If the length of the rectangle is denoted by L, and the width of the rectangle is denoted by W, then the formula for the perimeter (P) of the rectangle is:
P = 2L + 2W
So, to find the perimeter of a rectangle, you simply add up twice the length and twice the width of the rectangle.
The perimeter of a rectangle is the total distance around the outside of the rectangle. It is the sum of the lengths of all four sides of the rectangle. In other words, it is the measure of the distance that surrounds the shape or the length of the boundary.
The formula for the perimeter of a rectangle is:
P = 2(l + w)
Where "P" is the perimeter, "l" is the length of the rectangle, and "w" is the width of the rectangle.
For example, if a rectangle has a length of 5 units and a width of 3 units, then its perimeter can be calculated as:
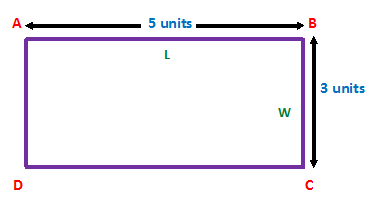
P = 2(5 + 3) = 2(8) = 16 units
Therefore, the perimeter of the rectangle is 16 units.