CLASS-4
DIVISIBILITY - PROBLEM & SOLUTION
DIVISIBILITY-PROBLEM & SOLUTION -
As we know so far the concept about divisibility, now we will learn the divisibility in more logical manner through problem solving.
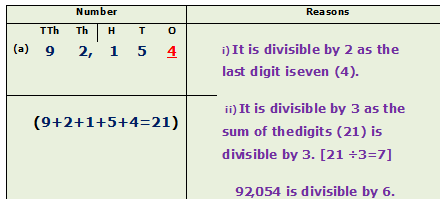
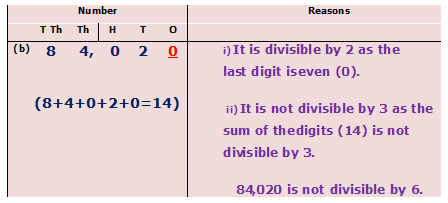
Example.1) Write whether the following numbers are divisible by 6 or not.
Give reasons: a. 92,154 b. 84,020
Ans.)
DIVISIBILITY BY 6 :-
EXPLANATION :
Ø Make a table as shown in example
Ø First, check divisibility by 2- underline the digit in the one’s place .
Ø If the underlined digit is even (0, 2, 4, 6, or 8), the given number is divisible by 2.
Ø Next, check divisibility by 3 - find the sum of the digits of the given number .
Ø If the sum of the digits is divisible by 3, the given number is divisible by 3.
Ø If the number is divisible both by 2 and 3, then the given number is divisible by 6.
Ø But, if the number is divisible by any one (either 2 or 3) or none, then the given number is not divisible by 6.
Example.2) Write the smallest number that should be (i) added to and (ii) subtracted from the following numbers to get them divisible by 6.
Ans.) (a) 81
(i) +3
3 should be added (81+3=84)
(ii) -3
3 should be subtracted (81-3=78)
EXPLANATION -
[Addition :-
It is not divisible by 2 as the last digit is not even (1).
If we add 1, it will be 82, but 8+2=10 which is not divisible by 3. 84 is the next even number, which is divisible both by 2 and 3.]
[For Subtraction :-
80 (not divisible by 3) and 79 (not divisible by 2).
But, 78 is divisible both by 2 and 3.]
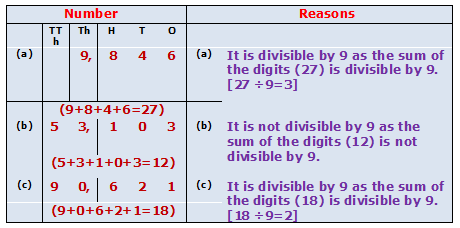
Example.3) Write whether the following numbers are divisible by 9 or not. Give reasons:
a) 9,846, b) 53,103, c) 90,621
Example.4) Write the smallest number that should be (i) added to and (ii) subtracted from the following numbers to get them divisible by 9.
(b) 277
(i) +2
2 should be added (277+2=279)
(ii) -7
7 should be subtracted (277-7=270)
EXPLANATION :-
[Addition :-
It is not divisible by 9 as the sum of digits is 16 (2+7+7=16). We know 18 is divisible by 9, we should add 2.
277+2 =279 is divisible by 9 (2+7+9=18). ]
[For Subtraction :-
2+7+7=16 is not divisible by 9.
16-7=9; 9 is divisible by 9.
We should subtract 7; 277-7=270
2+7+0=9; 270 is divisible by 9. ]
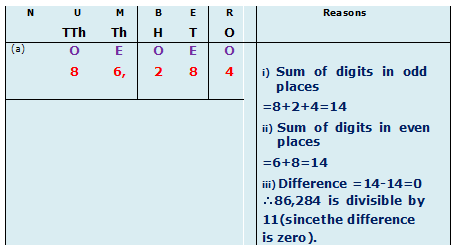
Example.5) Write whether the following numbers are divisible by 11 or not. Give reasons: a. 86,284 b. 7,441
Ans.)
DIVISIBILITY BY 11 :-
EXPLANATION :-
Ø Make a table as shown in example
Ø First, mark the odd and even places alternately in the table as shown. The first place is always odd.
Ø Find the sum of the digits that are in the odd places.
Ø Next, find the sum of the digits that are in the even places.
Ø Now, find the difference between these two sums (subtract).
Ø If the difference is exactly divisible by 11, the given number is divisible by 11.
Ø Or, if the difference is zero (0), the given number is divisible by 11.
Ø Otherwise the given number is not divisible by 11.
We should find sums of the digits in both odd and even places and their difference) [O=ODD PLACE ; E= EVEN PLACE]

Example.6) Write whether the following numbers are divisible by 12 or not. Give reasons. a. 23,940 b. 78,618
Ans.) DIVISIBILITY BY 12 :-
EXPLANATION :-
Ø Make a table as shown in example
Ø First, check divisibility by 3 - find the sum of the digits of the given number .
Ø If the sum of the digits is divisible by 3, the given number is divisible by 3.
Ø Next, check divisibility by 4 - underline the digits in the ones and tens places (i.e. the last two digits).
Ø If the underlined last two digits can be divided by 4 leaving no remainder, the given number is divisible by 4.
Ø OR, if the underlined last two digits are both zeroes (00), the given number is divisible by 4.
Ø If the number is divisible both by 3 and 4, then the given number is divisible by 12.
Ø But, if the number is divisible by any one (either 3 or 4) or none, then the given number is not divisible by 12.
CHECK DIVISIBILITY BY 3 and 4 BOTH

