CLASS-6
COEFFICIENT OF THE ALGEBRAIC TERM
COEFFICIENT OF THE ALGEBRAIC TERM -
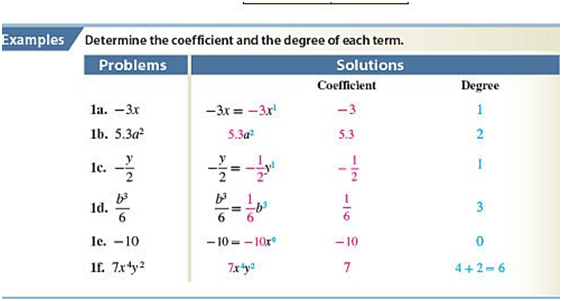
In algebra, the coefficient of an algebraic term is the numerical factor that multiplies the variables and their exponents within that term. Coefficients are constants, and they represent the magnitude or scale of the term. Coefficients can be positive, negative, or zero, and they provide valuable information about how the term contributes to the overall expression. Here are some examples of algebraic terms with their coefficients:
- Constant Term:- A constant term is a term that does not contain any variables. Its coefficient is simply the constant value itself.
In the term "5," the coefficient is "5."
2. Linear Term:- A linear term involves a single variable raised to the first power. The coefficient is the numerical factor in front of the variable.
In the term "3x," the coefficient is "3."
In the term "-2y," the coefficient is "-2."
3. Quadratic Term:- A quadratic term involves a single variable raised to the second power. The coefficient is the numerical factor in front of the variable raised to the second power.
In the term "2x²," the coefficient is "2."
In the term "-7y²," the coefficient is "-7."
4. Mixed Term:- A mixed term contains multiple variables raised to different powers. The coefficient is the numerical factor that multiplies the variables and their exponents.
In the term "4xy," the coefficient is "4."
In the term "-2xyz," the coefficient is "-2."
5. Negative Coefficients:- Coefficients can be negative, indicating a negative value.
In the term "-3x," the coefficient is "-3."
In the term "-0.5y," the coefficient is "-0.5."
6. Zero Coefficient:- Coefficients can also be zero, which means the term does not contribute to the overall value of the expression.
In the term "0 z³," the coefficient is "0," making the term equivalent to zero.
Understanding coefficients is essential when simplifying expressions, solving equations, or performing algebraic operations. Coefficients help determine how each term affects the overall behavior of the expression and play a significant role in analyzing and manipulating algebraic expressions and equations.