CLASS-6
COEFFICIENT & DEGREE
COEFFICIENT & DEGREE -
In algebra, the coefficient and degree are important attributes of a term within a polynomial expression. They provide valuable information about the term's behavior and role within the polynomial. Here's an explanation of both terms:
1. Coefficient:-
(i) The coefficient of a term is the numerical factor that multiplies the variables and their exponents within that term.
(ii) It is the constant part of the term and represents the magnitude or scale of the term.
(iii) The coefficient can be any real number, including positive, negative, or zero.
(iv) In a term of the form "a xⁿ," where "a" is the coefficient, "x" is the variable, and "n" is the exponent, "a" is the coefficient.
Examples:-
(a.) In the term "3x²," the coefficient is "3."
(b.) In the term "-2y," the coefficient is "-2."
(c.) In the term "7," the coefficient is "7" (a constant term with no variables).
2. Degree:-
(i) The degree of a term is determined by the exponents of the variables within that term.
(ii) It is the highest power to which any variable within the term is raised.
(iii) If a term contains no variables, its degree is considered to be 0.
(iv) In a term of the form "a xⁿ," where "a" is the coefficient, "x" is the variable, and "n" is the exponent, "n" is the degree.
Examples:-
(a.) In the term "3x²," the degree is "2" because "x" is raised to the second power.
(b.) In the term "-2y," the degree is "1" because "y" is raised to the first power.
(c.) In the term "7," the degree is "0" (a constant term with no variables).

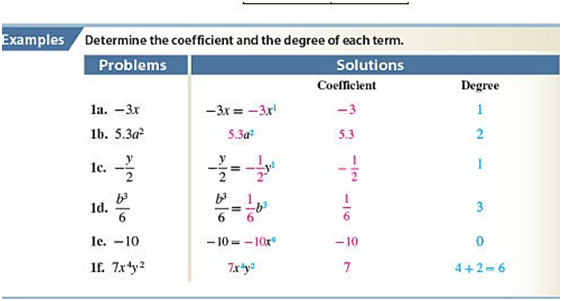
It's important to note that the degree of a polynomial is determined by the highest degree term within that polynomial. The degree of the polynomial is the same as the degree of its highest degree term.
For example, in the polynomial expression "3x³- 2x²+ 4x - 7," the degree is 3 because the term "3x³" has the highest degree (exponent 3). The coefficient of this term is 3.
Understanding coefficients and degrees is essential in polynomial algebra, as they help classify polynomials, analyze their behavior, and determine various properties of polynomial expressions.