CLASS-6
MULTIPLICATION OF ALGEBRAIC EXPRESSIONS IN MONOMIAL

MULTIPLICATION OF ALGEBRAIC EXPRESSIONS IN MONOMIAL -

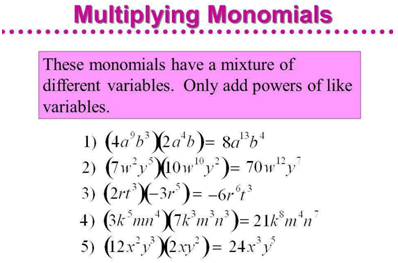
Multiplying algebraic expressions that consist of monomials is relatively straightforward. A monomial is a single term that may include variables raised to integer exponents and coefficients. To multiply two monomials together, you can follow these general steps:

1. Multiply the coefficients:- Multiply the numerical coefficients (numbers in front of the variables) of the two monomials.
2. Multiply the variables:- If the monomials have variables with the same base, add the exponents of those variables together. If the bases are different, leave them as separate terms.
3. Combine like terms:- If there are multiple terms with the same variable base, combine them by adding or subtracting their coefficients.

Here's an example to illustrate these steps:-

Example.1) Let's multiply the monomials 3x² and 4x³.

1. Multiply the coefficients:- 3 * 4 = 12.
2. Multiply the variables:-  For the variable x, add the exponents: 2 + 3 = 5. So, you have x⁵.
3. Combine like terms:- There are no other terms with the same variable base, so you're done.

The product of 3x² and 4x³ is 12x⁵.           (Ans.)

Here's another example with different bases:-

Example.2) Multiply 2a³ and 5b².

1. Multiply the coefficients:-  2 * 5 = 10.
2. Multiply the variables:-  For the variable a, the exponent is 3. For the variable b, the exponent is 2.
3. Combine like terms:-  There are no other terms to combine because a and b are different variables.

The product of 2a³ and 5b² is 10a³b².      (Ans.)