CLASS-6
MULTIPLICATION OF ALGEBRAIC EXPRESSION OF BINOMIAL BY BINOMIAL
MULTIPLICATION OF ALGEBRAIC EXPRESSION OF BINOMIAL BY BINOMIAL -
When multiplying two binomials (algebraic expressions with two terms each), you can use the FOIL method, which stands for First, Outer, Inner, Last. This method helps you remember the order in which to multiply the terms in each binomial and combine them to obtain the result. Here are the steps:
Let's multiply two binomials:- (A + B) and (C + D).
1.) First: Multiply the first terms of each binomial.
(A + B) (C + D)
First:- A * C = AC
2.) Outer: Multiply the outer terms of each binomial.
(A + B) (C + D)
Outer:- A * B = AB
3.) Inner: Multiply the inner terms of each binomial.
(A + B) (C + D)
Inner:- B * C = BC
4.) Last: Multiply the last terms of each binomial.
(A + B) (C + D)
Last:- B * D = BD
5.) Combine the results: Add all four results together.
Now, combine these four products together to obtain the final result:
Result = (A * C) + (A * D) + (B * C) + (B * D)
So, the product of (A + B) and (C + D) is:
Result = AC + AD + BC + BD
So, (A + B) (C + D) = AC + AD + BC + BD
Here's a concrete example:-
Example.1) Multiply (3x + 2) and (4x - 5).
Ans.)
Using the FOIL method:-
- First:- (3x) * (4x) = 12x²
- Outer:- (3x) * (-5) = -15x
- Inner:- (2) * (4x) = 8x
- Last:- (2) * (-5) = -10
Now, combine these products:
Result = 12x²- 15x + 8x - 10
Finally, combine like terms:
Result = 12x²- 7x - 10
So, the product of (3x + 2) and (4x - 5) is 12x²- 7x - 10. (Ans.)
Example.2) Multiply (x + 3) (x + 2)
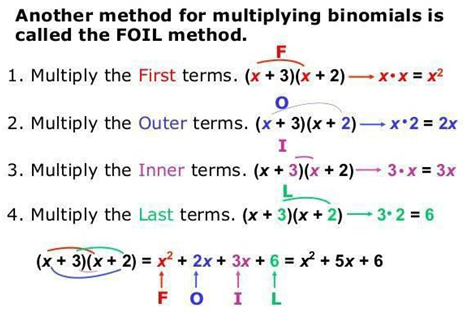
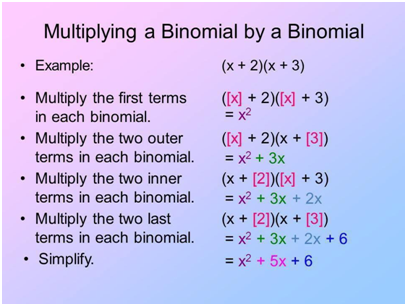
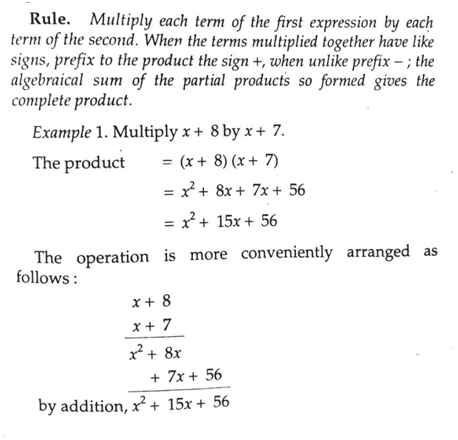
Example.3) Multiply (x + 8) (x + 7)
Example.4) Multiply (5a - 6b) (7a + 8b)
