CLASS-6
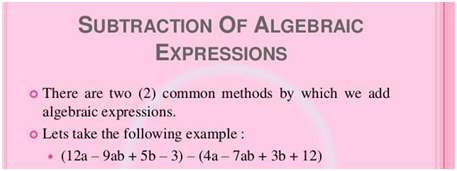
SUBTRACTION OF ALGEBRAIC EXPRESSION

SUBTRACTION OF ALGEBRAIC EXPRESSION -

Subtracting algebraic expressions involves subtracting one expression from another. When subtracting algebraic expressions, you should follow these steps:

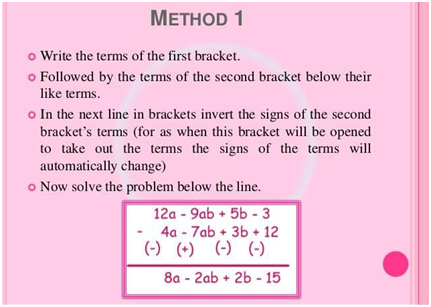
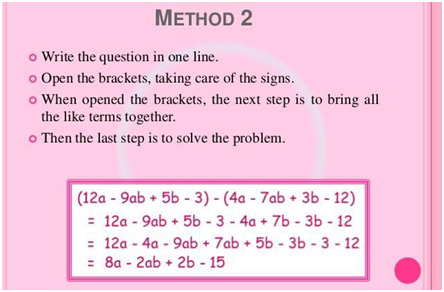
1. Distribute the Subtraction Sign:- Distribute the subtraction (negative) sign to every term within the expression being subtracted. This ensures that you subtract each term correctly.
2. Organize the Terms:- Write down the expressions, with the subtraction sign distributed, so you can see the terms you'll be subtracting.
3. Combine Like Terms:- Within each expression, combine the like terms by subtracting their coefficients. Like terms have the same variables with the same exponents.
4. Retain the Variable Part:- Keep the variable parts, including their exponents, unchanged. The variable part represents the common variable(s) shared by the like terms.
5. Perform the Subtraction:- Perform the subtraction for each group of like terms or constants separately.
6. Write the Result:- Express the final result as the difference of the combined terms and any remaining terms.

Here's an example of subtracting algebraic expressions:

Example.1) Subtracting Algebraic Expressions

Given the expressions:

• Expression A:-   4x²- 3xy + 2y
• Expression B:-   2x²+ 5xy - y

Distribute the subtraction sign and organize the terms.

Ans.)

• Expression A - Expression B = (4x²- 3xy + 2y) - (2x²+ 5xy - y)

Combine like terms separately:

• (4x²- 2x²) - (3xy - 5xy) + (2y + y)

Now, subtract the like terms:

• 2x²- (-2xy) + 3y

Combine the coefficients for like terms:

• 2x²+ 2xy + 3y

So, the simplified result of subtracting the algebraic expressions is 2x²+ 2xy + 3y.

In this example, we distributed the subtraction sign, organized the terms, combined like terms separately, and then expressed the final result as the difference of the combined terms.