CLASS-6
BISECTOR OF AN ANGLE

BISECTOR OF AN ANGLE -

The bisector of an angle is a line or ray that divides the angle into two equal parts. Specifically, if you have an angle formed by two rays or line segments, the bisector is a line (or ray) that divides that angle into two smaller angles, and each of these smaller angles is equal in measure.

There are a few ways to construct the bisector of an angle:-

1. Angle Bisector Theorem:- If you have triangle ABC with angle ∠BAC, the angle bisector of ∠BAC divides the opposite side BC into segments in such a way that the ratio of the length of AB to the length of AC is equal to the ratio of the length of the two segments into which the angle bisector divides side BC.
2. Compass and Straightedge Construction:- To construct the angle bisector of a given angle, you can use a compass and a straightedge. Here's a basic outline of the construction:- (1) Place the compass point at the vertex of the angle. (2) Draw two arcs that intersect each side of the angle. (3) Where these arcs intersect, draw a straight line from the vertex to this intersection point. This line is the angle bisector.
3. Protractor Measurement:-  If you know the angle measure, you can use a protractor to measure half of the angle, and then draw a line from the vertex through the point where the protractor bisected the angle.

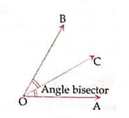
A line that divides an angle into two equal parts is called a bisector of an angle. 

In the adjoining diagram, ∠AOC = ∠COB,

So, OS is bisector of ∠AOB.

Remember that the angle bisector divides the angle into two angles of equal measure. This concept is often used in geometry and trigonometry.