CLASS-6
TRANSVERSAL ANGLE
TRANSVERSAL ANGLE -
A transversal is a line that intersects two or more other lines at distinct points. The term "transversal" is commonly used in geometry, and the intersection of the transversal with other lines creates specific angle relationships. Here are some key points about transversals:-
(1) Definition -
A transversal is a line that crosses or intersects two or more lines.
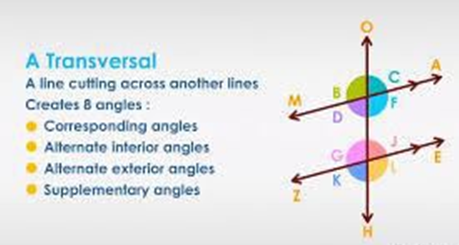
(2) Angle Relationship -
- When a transversal intersects a pair of parallel lines, it creates several types of angles, including corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles. These angles have specific relationships based on the positions of the angles with respect to the parallel lines and the transversal.
(3) Corresponding Angles:-
- Corresponding angles are angles that occupy the same relative position at each intersection on the same side of the transversal. When the lines are parallel, corresponding angles are congruent (equal).
(4) Alternate Interior Angles:-
- Alternate interior angles are angles located on opposite sides of the transversal and inside the parallel lines. When the lines are parallel, these angles are congruent.
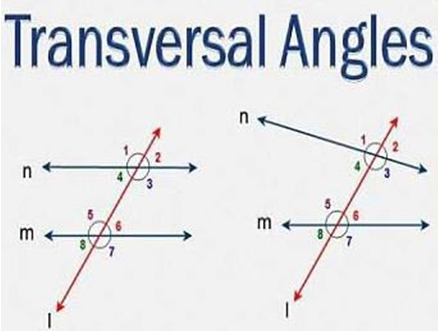
(5) Alternate Exterior Angles:-
- Alternate exterior angles are angles located on opposite sides of the transversal and outside the parallel lines. They are congruent when the lines are parallel.
(6) Consecutive Interior Angles (Same-Side Interior Angles):-
- Consecutive interior angles are angles located on the same side of the transversal and inside the parallel lines. They are supplementary, meaning their measures add up to 180 degrees when the lines are parallel.
Transversals play a crucial role in geometry, particularly when dealing with parallel lines. Understanding the angle relationships formed by transversals is fundamental for solving geometric problems and proofs involving parallel lines and angles.