CLASS-6
TRIANGLE - OBTUSE TRIANGLE

OBTUSE TRIANGLE -

An obtuse triangle is a type of triangle in which one of the interior angles is greater than 90 degrees, making it an obtuse angle. In other words, an obtuse triangle is a triangle that contains one angle larger than a right angle (90 degrees) and two acute angles (less than 90 degrees).

Key features of an obtuse triangle:-

  1. Obtuse Angle:- There is at least one angle in the triangle that measures more than 90 degrees.
  2. Other Angles:- The other two angles are acute, meaning they are less than 90 degrees.
  3. Sum of Angles:- The sum of the interior angles in any triangle is always 180 degrees. In an obtuse triangle, the sum is accounted for by one angle being greater than 90 degrees.

Here's an illustration of an obtuse triangle:-

Geometry

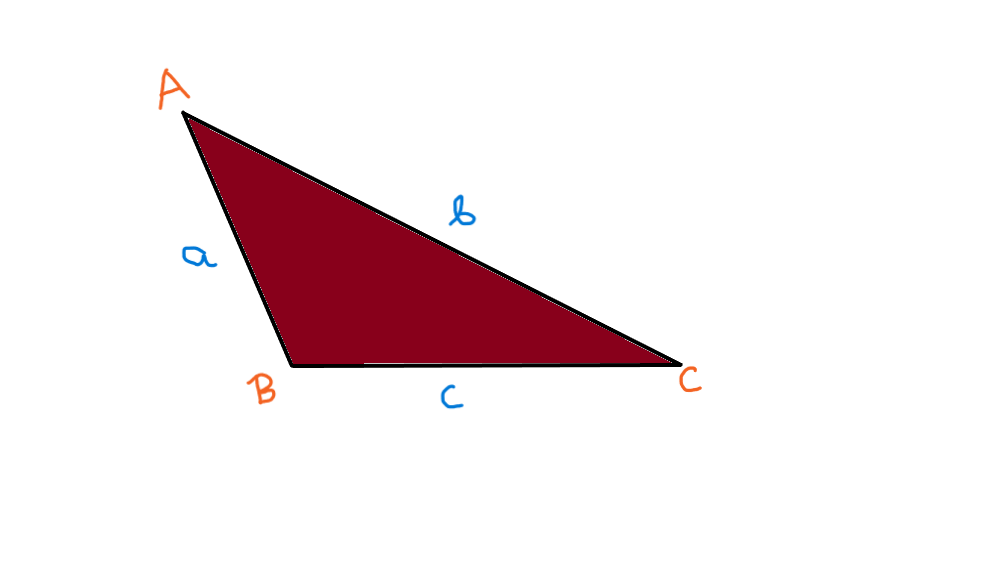
In the above triangle ABC:-

  • Angle B is the obtuse angle.
  • Angle A and Angle C are acute angles.
  • Side lengths are denoted as "a," "b," and "c."

Obtuse triangles can have various side lengths and configurations. They are a common geometric shape, and understanding their properties is important in geometry and trigonometry. The classification of triangles into types, such as acute, right, and obtuse, is based on the measures of their angles.