CLASS-6
ADDITION OF INTEGERS
ADDITION OF INTEGERS -
The addition of integers involves combining two or more integers to find their total or sum. Adding integers is a fundamental arithmetic operation and follows specific rules based on the signs of the integers being added. Let's go through the rules and examples of adding integers:
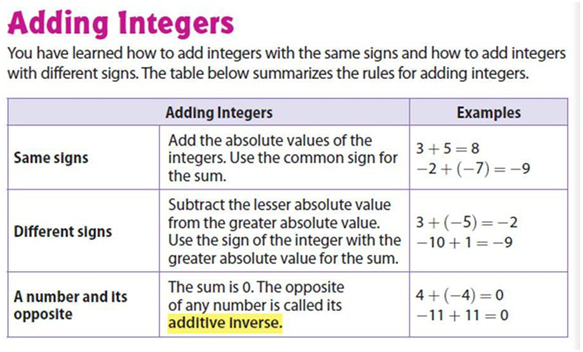
1. Adding Positive Integers:- When you add two positive integers, you simply add their numerical values. Example: 3 + 5 = 8.
Start at the initial point (usually 0) on the number line and move to the right (in the positive direction) by the value of the positive integer you want to add.
Example: 3 + 4 Starting at 0, move 3 units to the right to reach 3. Then, move 4 more units to the right to reach the final point at 7.
0 1 2 3 4 5 6 7
↑------↑
2. Adding Negative Integers:- When you add two negative integers, you also add their numerical values, and the result is negative. Example: -4 + (-2) = -6.
Start at the initial point (usually 0) on the number line and move to the left (in the negative direction) by the absolute value of the negative integer you want to add.
Example: -2 + (-5) Starting at 0, move 2 units to the left to reach -2. Then, move 5 more units to the left to reach the final point at -7.
-7 -6 -5 -4 -3 -2 -1 0 1 2 3 4 5 6 7
↑-------------↑
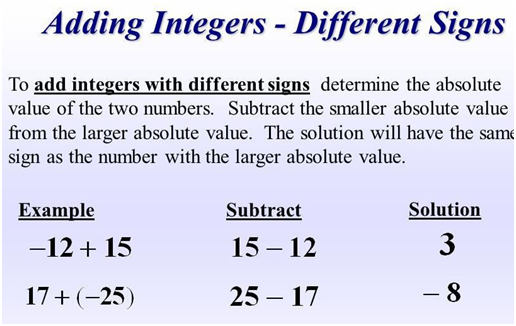
3. Adding a Positive and a Negative Integers:- When you add a positive integer and a negative integer, subtract the smaller absolute value from the larger absolute value, and the sign of the result is determined by the integer with the larger absolute value. Example: 7 + (-3) = 7 - 3 = 4
Start at the initial point (usually 0) on the number line. Move to the right by the absolute value of the positive integer, and then move to the left by the absolute value of the negative integer.
Example: 3 + (-2) Starting at 0, move 3 units to the right to reach 3. Then, move 2 units to the left to reach the final point at 1.
-2 -1 0 1 2 3 4 5
↑----↑↑------↑
4. Zero Property:- Adding zero to any integer doesn't change the value of that integer. Example: 9 + 0 = 9
Here are a few more examples to illustrate these concepts:
- 2 + 3 = 5 (Positive + Positive)
- -5 + (-7) = -12 (Negative + Negative)
- 8 + (-4) = 8 - 4 = 4 (Positive + Negative)
- -3 + 6 = 6 - 3 = 3 (Negative + Positive)
- 0 + 10 = 10 (Adding zero)
Keep in mind that the number line can be a helpful visual tool to understand addition of integers. When adding integers with different signs, think of moving to the right (positive direction) on the number line for positive numbers and moving to the left (negative direction) for negative numbers. The distance you move corresponds to the numerical value of the integer.
Remember that when adding integers, you can always think of positive numbers as moving to the right on the number line and negative numbers as moving to the left. The distance you move represents the absolute value of the integer you're adding. The final point you reach gives you the sum of the integers.
Using the number line as a visual aid can help you better understand the concept of adding integers, especially when dealing with different signs and magnitudes. These rules and practice with more examples to develop a solid understanding of adding integers.