CLASS-7
RECURRING DECIMALS
RECURRING DECIMALS:-
A recurring decimal (also called a repeating decimal) is a decimal fraction that has a digit or a sequence of digits that repeats infinitely. These decimals can be represented in two ways:
- As a bar notation:- For example, 0.333… is written as 0.3‾, where the bar ( ‾ ) indicates that the digit 3 repeats indefinitely.
2. As a fraction:- Every recurring decimal can be expressed as a fraction of two integers (a rational number).
1 4
For example, 0.3‾= ------ and 0.12‾= -------
3 33
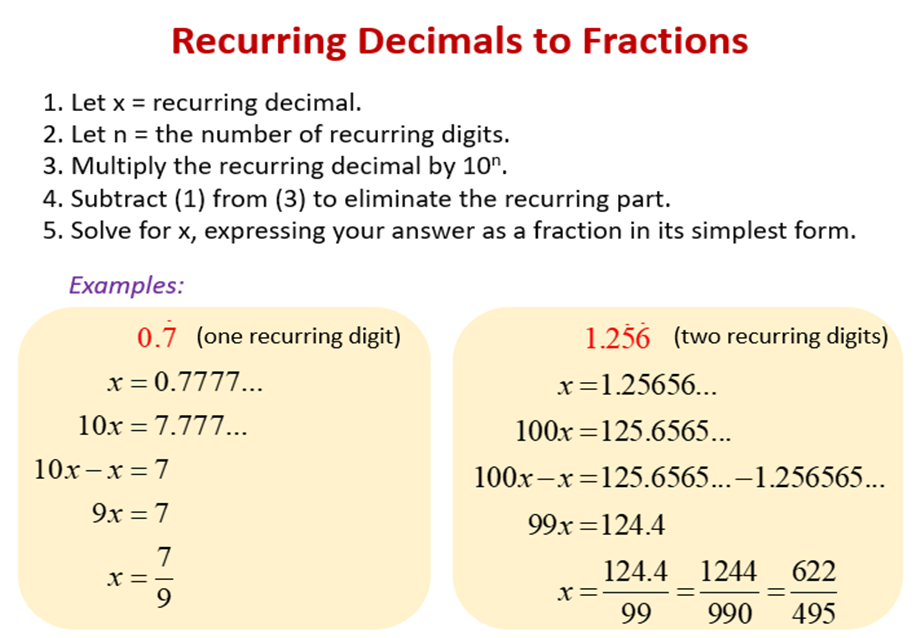
How to Convert a Recurring Decimal to a Fraction:-
Here's the general process for converting:
Example 1: Single Digit Repeats (0.6‾)
Ans.)
Step.1:- Let x = 0.6‾.
Step.2:- Multiply both sides by 10 to shift the repeating part:
10x = 6.6‾ X 10
Step.3:- Subtract the original equation:
(10x − x) = (6.6‾− 0.6‾)
6 2
Step.4:- Solve for x = ------ = ------
9 3
Example 2: Multiple Digits Repeat (0.123‾)
Ans.)
Step.1:- Let x = 0.123‾
Step.2:- Multiply by 10ⁿ, where n is the number of repeating digits (here n = 3):
1000x = 123.123‾
Step.3:- Subtract the original equation:
1000x − x = 123.123‾ − 0.123‾
giving, 999x = 123
123 41
Step.4:- Solve for x = -------- = -------
999 333