CLASS-7
SURFACE AREA AND VOLUME OF CUBOID
SURFACE AREA AND VOLUME OF CUBOID -
A cuboid (also known as a rectangular prism) is a three-dimensional solid object with six rectangular faces, where opposite faces are equal. It has length (l), width (w), and height (h) as its dimensions.
1. Surface Area of a Cuboid:-
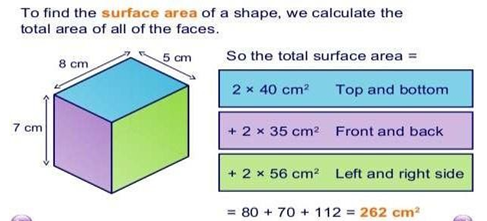
The surface area is the total area of all six rectangular faces.
A = 2lw + 2lh + 2wh
Where:-
- l = length
- w = width
- h = height
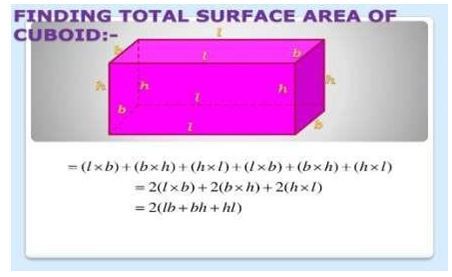
You are essentially summing the areas of three pairs of opposite faces:-
- 2lw for the front and back faces,
- 2lh for the top and bottom faces, and
- 2wh for the left and right faces.
Surface area of a cuboid = 2 (lb+ bh+ lh)
Example.1) If the length, breadth and height of a cuboid are 5 cm, 3 cm and 4 cm. The find its total surface area.
Ans.)
Given, Length, l = 5 cm,
Breadth, b = 3 cm
and Height, h = 4 cm.
As per the formula -
TSA = 2(lb+bh+lh)
TSA = 2(5 x 3 + 3 x 4 + 5 x 4)
TSA = 2(15 + 12 + 20)
TSA = 2(47) = 94 sq.cm.
Volume Of Cuboid:-
The volume is the amount of space enclosed by the cuboid.
V= lwh
Where:
- l, w, and h are the dimensions of the cuboid.
This formula calculates the volume by multiplying the area of the base (length × width) by the height.

Example.1) Find the volume of a cuboid of length 20 cm, breadth 15 cm and height 10 cm.
Ans.)
Length of the cuboid = 20 cm Breadth of the cuboid = 15 cm Height of the cuboid = 10 cm
Therefore, volume of the cuboid = length × breadth × height
= (20 × 15 × 10) cm³
= 3000 cm³ (Ans.)
Example.2) If a cuboid has dimensions:
- Length l = 5 cm,
- Width w = 3 cm,
- Height h = 4 cm,
Then:
- Surface Area:-
A = 2(5×3) + 2(5×4) + 2(3×4)
= 2(15) + 2(20) + 2(12)
= 30 + 40 + 24
= 94 cm²
- Volume:-
V = 5cm × 3cm × 4cm = 60 cm³ (Ans.)