CLASS-7
FINDING SQUARE ROOT OF FRACTION
FINDING SQUARE ROOT OF FRACTION -
Method 1:- Find Square Root of Numerator and Denominator Separately
If both the numerator and denominator are perfect squares, simply take their square roots separately.
Example 1: Find √(49/64)
- √49 = 7
- √64 = 8
- Answer: 7/8 (Ans.)
Method 2:- Convert to a Decimal and Use Long Division
If the fraction is not a perfect square, convert it to a decimal and then find its square root using the long division method.
Example 2: Find √(5/8)
- Convert to decimal:- 5/8 = 0.625
- Use the long division method to find √0.625 (similar to finding the square root of a decimal).
- The approximate square root is 0.79.
Method 3:- Use Square Root Property
√a
For any fraction a/b, √ab = -------
√b
If the denominator is not a perfect square, multiply the fraction by a value that makes the denominator a perfect square.
Example 3: Find √(2/5)
√2
- Rewrite as: --------
√5
√5 √2 x √5 √10
2. Multiply by ------- to rationalize ----------- = --------
√5 √5 x √5 5
3. Approximate:-
√10 ≈ 3.162
√10 3.162
--------- = --------- ≈ 0.632
5 5
5
Example 4) Find the square root of 6 ------ correct to 2 decimal
8
places.
Ans.)
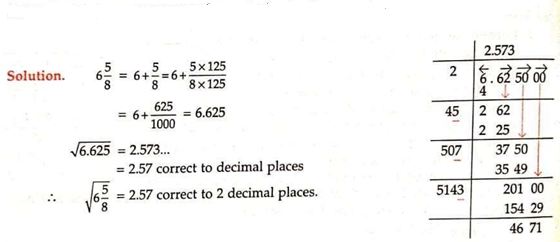
So, the answer is 2.57 (Ans.)
2
Example 5) Find the square root of 20 ------- correct to 2
3
decimal places.
Ans.)

So, the answer is 4.55 (Ans.)