CLASS-8
GEOMETRY-TRIANGLE-INEQUALITIES-IN-A-TRIANGLE

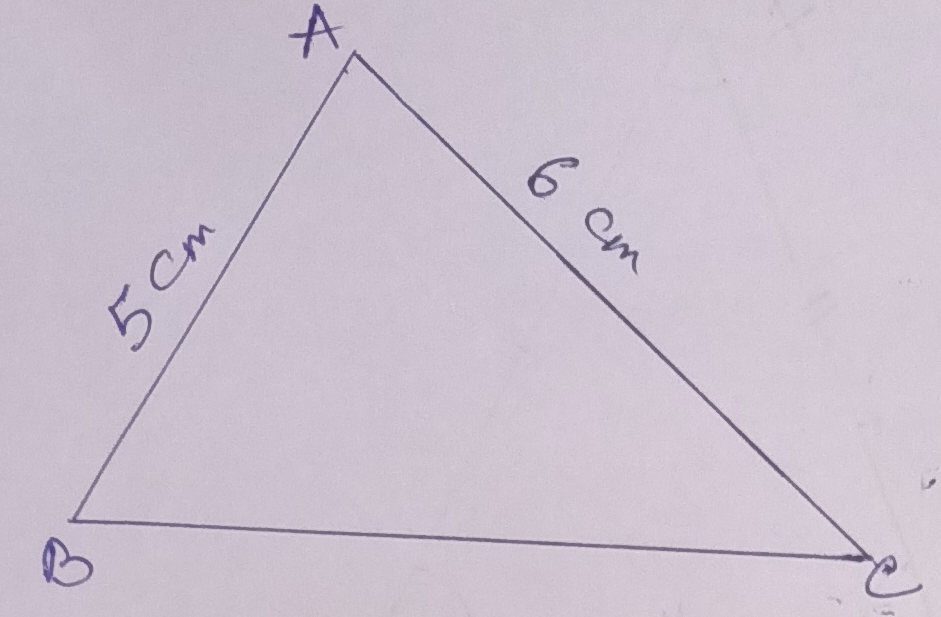
Inequality 1) –

If two sides of a triangle are considered unequal, then the greater side has the greater angle just opposite to it.

In the figure, AC > AB,

SO, ∠ABC > ∠ACB

Converse -

In a triangle, the side just opposite to the greater angle is considered to be greater

In the figure, ∠ABC > ∠ACB,

So,  AC > AB

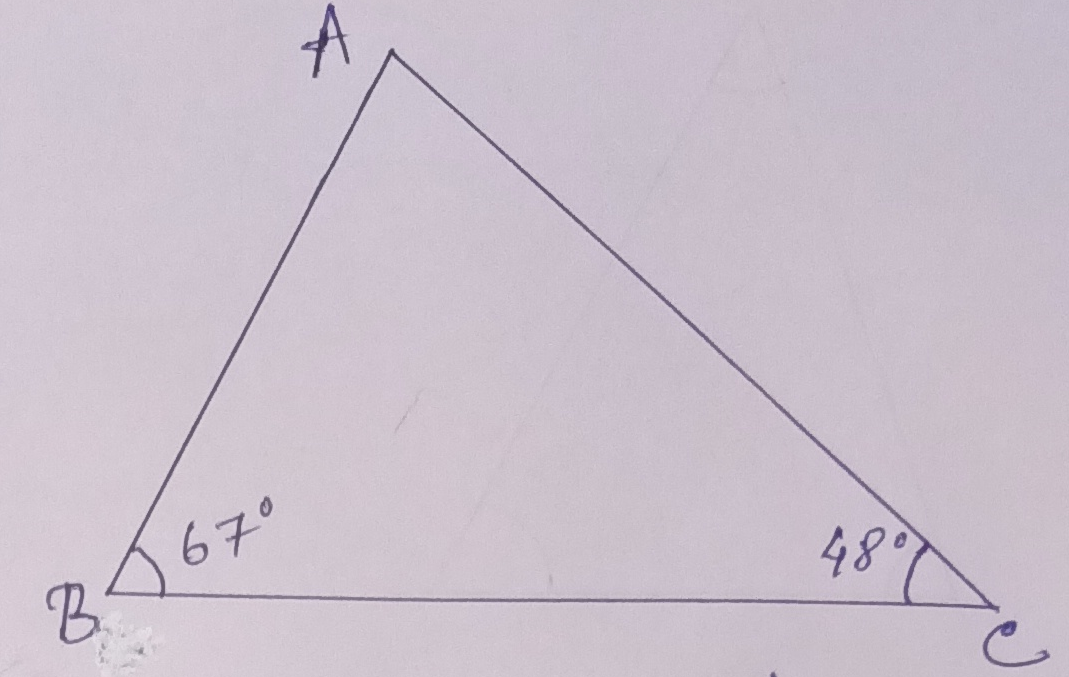
Inequality 2) –

Any two sides of any triangle that are together will be considered greater than the third side of the triangle.

In other words, in any ∆ ABC,   AB + AC > BC,

                                                     BC + BA > CA,

                                                     CA + CB > AB

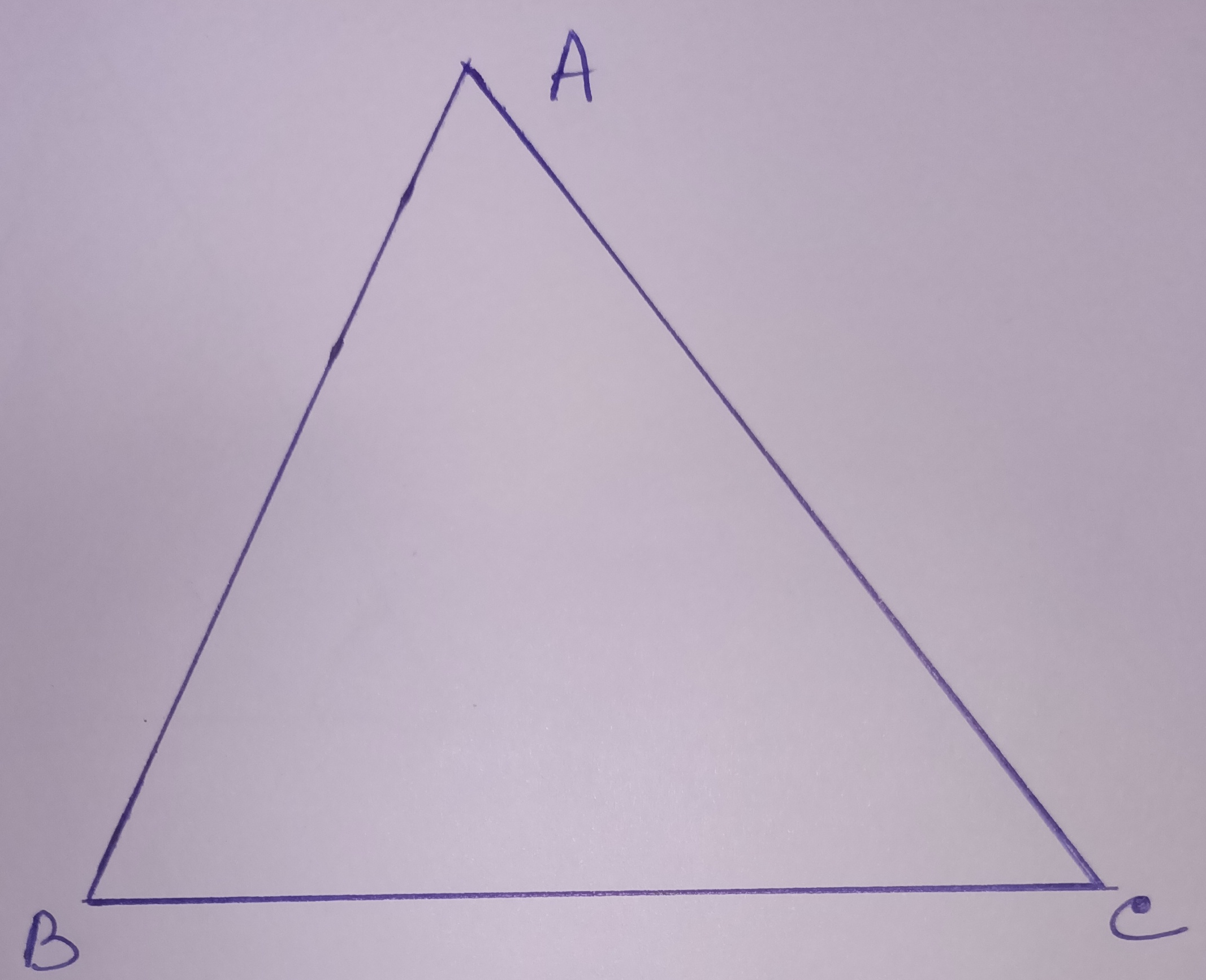
Inequality 3) –

The difference between any two sides of a triangle is considered smaller than the third side of the said triangle.

In the figure, AC – AB < BC,

              BC – AC < AB,

              BC – AB < AC