CLASS-8
PROBLEM & SOLUTION OF CONGRUENT 

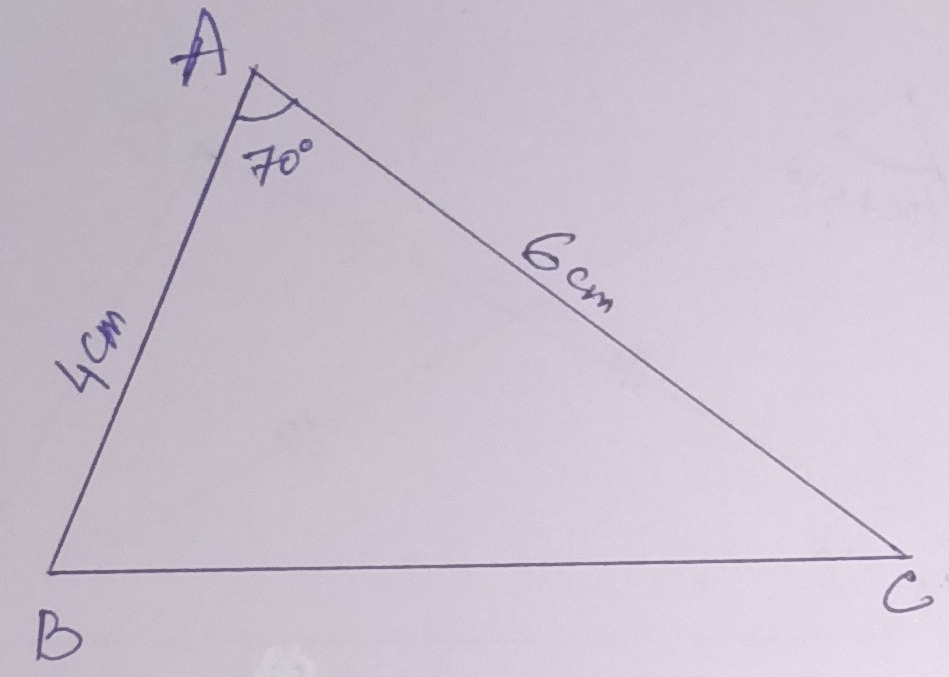
Ans.)  In ∆ DEF,

∠DEF = 180⁰ - 100⁰ =  80⁰

∠EFD = 180⁰ - 150⁰ =  30⁰

 So, ∠EDF =  180⁰ - (80⁰ + 30⁰) =  70⁰

In, ∆ ABC & ∆ DEF, AB = DE, ∠BAC =  ∠EDF  (= 70⁰) and AC = DF.

So, the S-A-S condition of congruence is satisfied.

So, ∆ ABC ≅ ∆ DEF

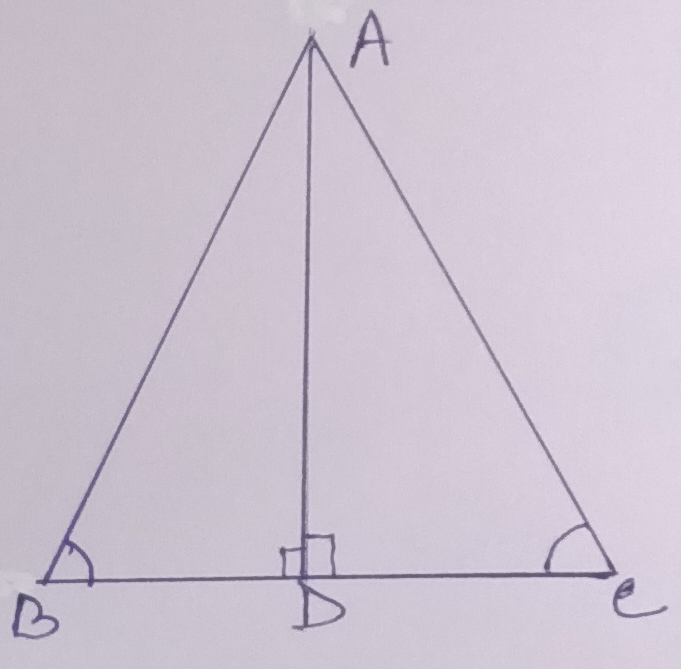
Ans.)

1) given, ∠ABD = ∠ACD => AC = AB

In ∆ ABD and ∆ ACD, ∠ABD = ∠ACD,  ∠ADB = ∠ADC (= 90⁰) and AB = AC .

So, A-A-S condition of congruence is satisfied.

∆ ABD ≅  ∆ ACD

2) the corresponding parts of ∆ ABD and  ∆ ACD are equal

So, BD = CD, that is, D is the mid-point of BC

3) also,  ∠BAD = ∠CAD, that is, AD bisects ∠BAC

Example.3) In the adjoining figure, AB bisects ∠CAD and  AC = AD, prove that (a)  ∆ ABC ≅  ∆ ABD and (b) BC = BD

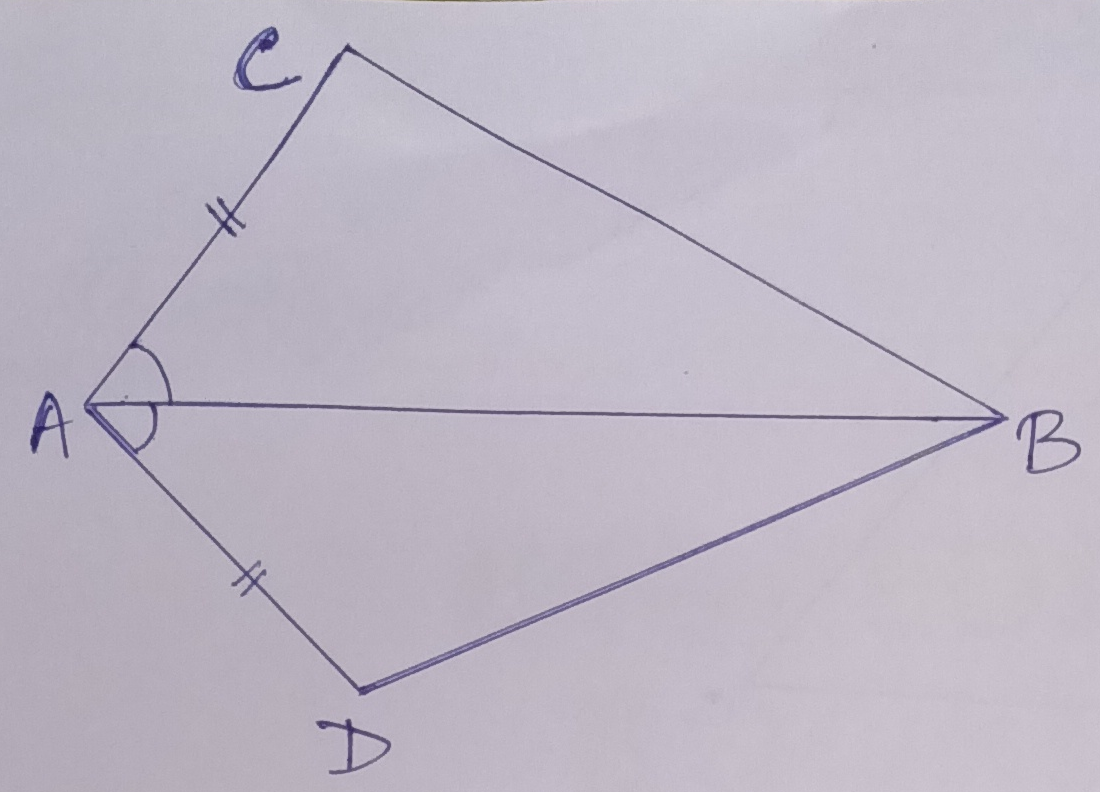
Ans.)  ∆ ABC and ∆ ABD, 

AC = AD  (given),

      ∠BAC = ∠BAD              (where AB bisects ∠CAD )

       AB = AB

So, the S-A-S condition is satisfied.

So,   ∆ ABC ≅ ∆ ABD

(b) the corresponding sides of ∆ ABC and ∆ ABD are equal. 

    So , BC = BD

Example.4) In the adjoining figure, prove that – (a) ∆ DBC ≅  ∆ EAC and  (b)  DC = EC

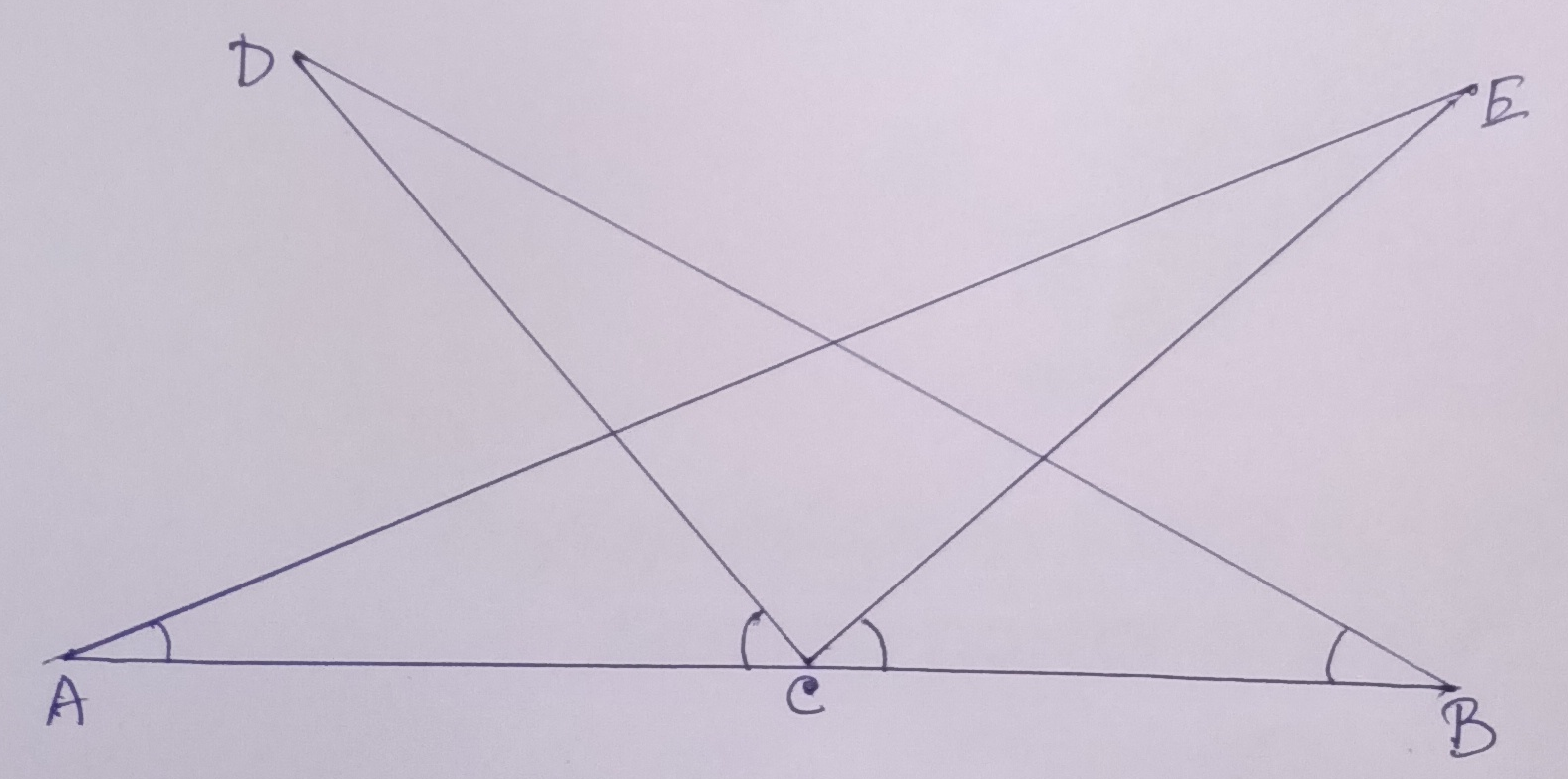
Ans.) As per the given condition, ∠ECB = ∠DCA

 Adding, ∠DCE to both sides,

∠ECB + ∠DCE = ∠DCA + ∠DCE

  =>   ∠DCB  =  ∠ECA ………………………. (a)

In ∆ DBC and  ∆ EAC, ∠DCB = ∠ECA   [using (a)],  BC = AC   (given)

And,  ∠DBC =  ∠EAC        (given)

So,  the A-S-A condition is satisfied,

So,    ∆ DBC ≅ ∆ EAC.

The corresponding sides of ∆ DBC and ∆ EAC are equal.

So,    DC = EC …………………………………….(b)

Example.5) In the adjoining figure, AB = CD and  AB || CD. Prove that –

a)  ∆ AOB ≅  ∆ DOC and

b)  AD & BC bisect each other at the point ‘0’

Ans.)  AB || CD => ∠OAB = alternate ∠ODC and ∠OBA = alternate ∠OCD

a) In ∆ AOB  and  ∆ DOC,

∠OAB = ∠ODC,

∠OBA = ∠OCD and AB = CD

so, the A-S-A condition of congruence is satisfied.

  So,  ∆ AOB ≅  ∆ DOC

b)  so, the corresponding sides of ∆ AOB and  ∆ DOC are equal.

   So, AO = DO and BO = CO

Hence, AD & BC bisect each other at the point O

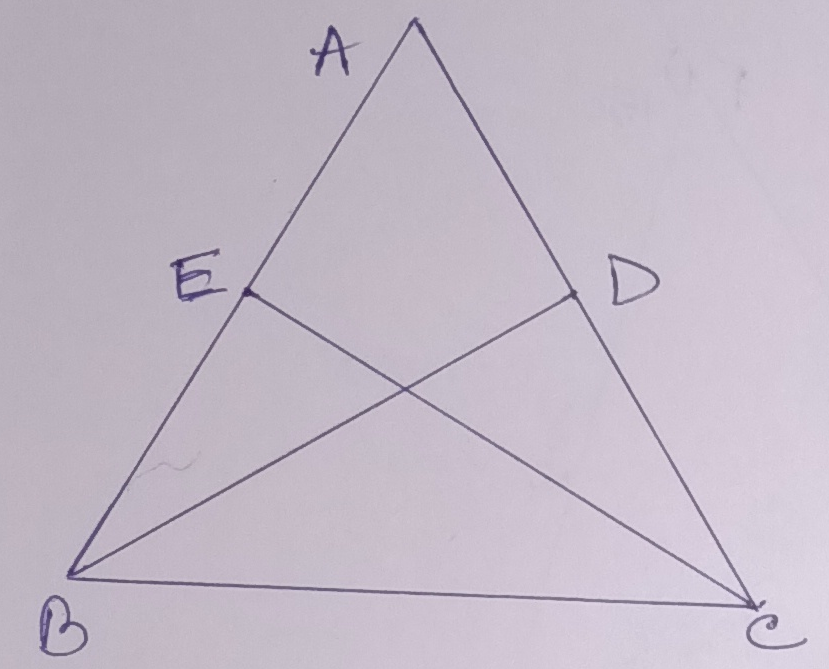
Example.6)  In the adjoining figure figure, BD = CE and  ∠ADB = 90⁰ = ∠AEC, prove that –

a) ∆ ABD ≅  ∆ ACE and

b) ABC is an isosceles triangle in which AB = AC

Ans.) 

a)  In  ∆ ABD and  ∆ ACE, ∠ADB = ∠AEC   (=90⁰)    (given)

∠BAD = ∠CAE and BD = CE (given)

The A-A-S condition of congruence is satisfied.

  So,  ∆ ABD ≅ ∆ ACE

b)  The corresponding sides of ∆ ABD and  ∆ ACE  are equal.

So,  AB = AC, that is, ABC is an isosceles triangles

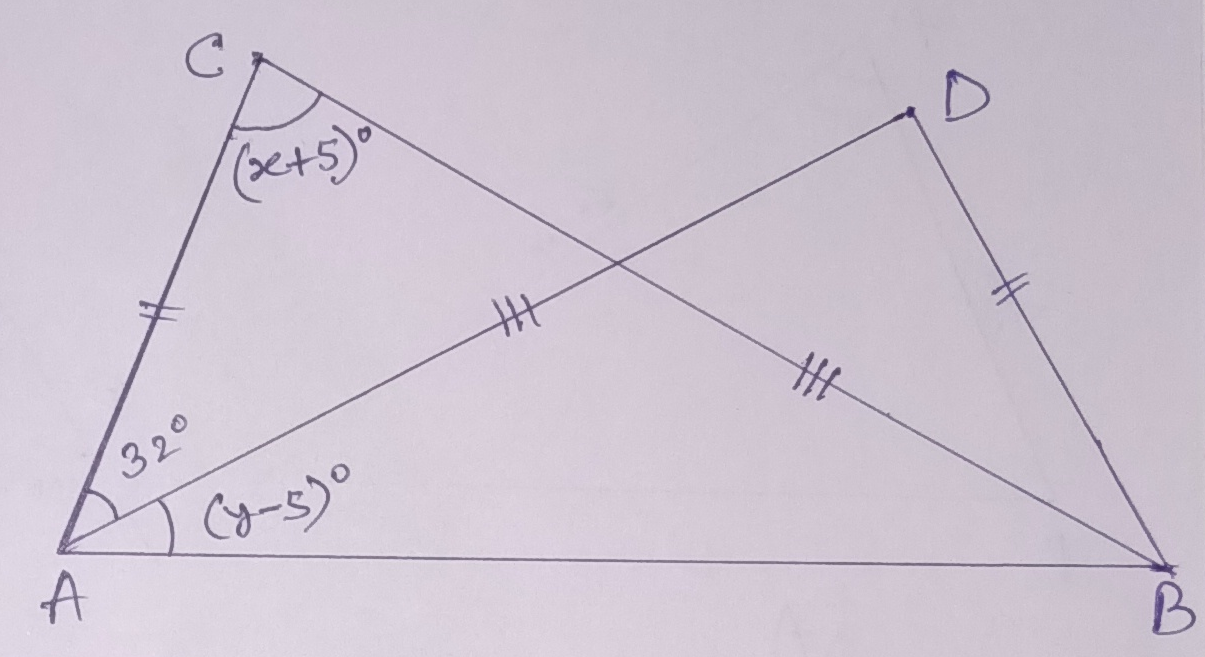
Example.7) Find x & y in each of the following figure.

Ans.) 

a) In ∆ CAB and ∆ DBA, AC = BD  (given),

  BC = AD    (given)

And, AB = AB

So, ∆ CAB ≅  ∆ DBA        (S-S-S condition)

So,  ∠ACB = ∠BDA,   ∠CBA = ∠DAB = (y – 5)⁰ and ∠CAB = ∠DBA,

      x + 5 = 64    or,   x  =  59

in, ∆ ABC, (x + 5)⁰ + 32⁰ + (y – 5)⁰ + (y – 5)⁰ =  180⁰

now,      59 + 5 + 32 + 2y – 10 = 180

or,         2y =  180 – 86  =  94

or,          y  =  94 / 2  =  47 

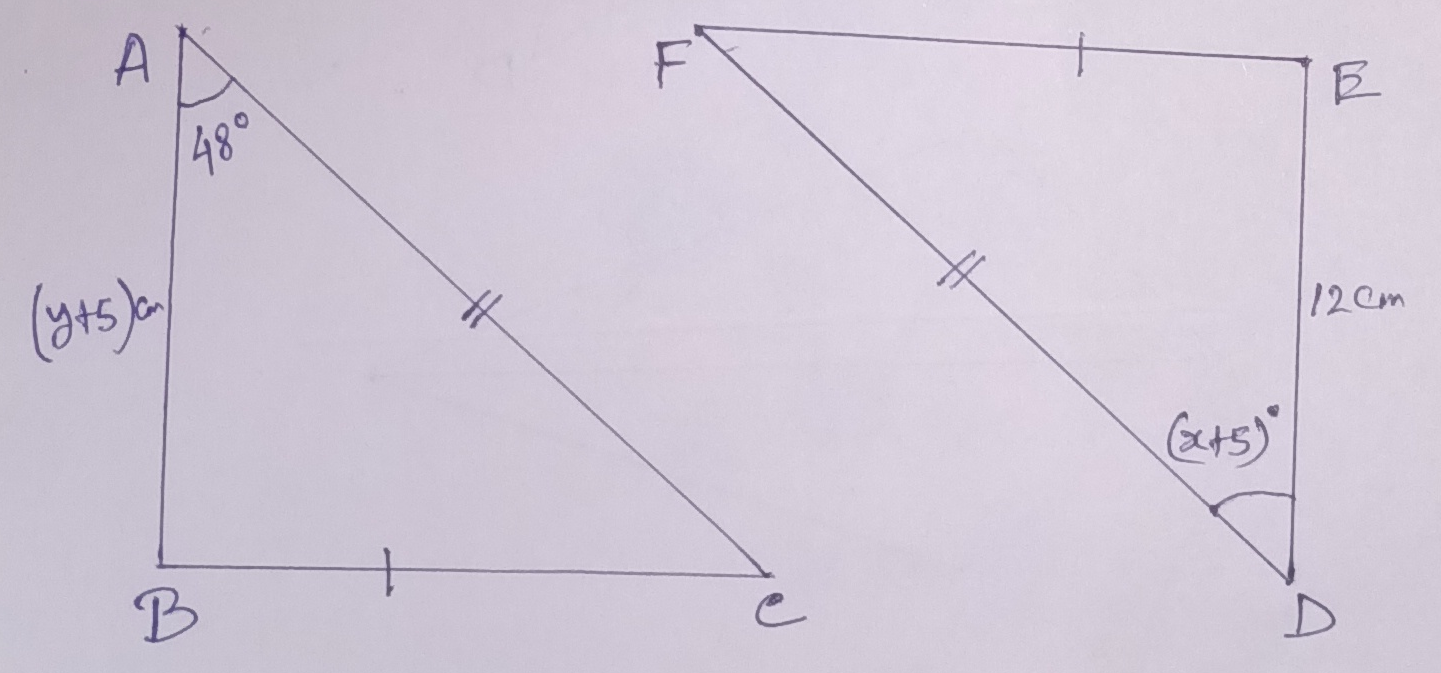
b)  In, ∆ ABC and ∆ DEF,  ∠ABC = ∠DEF  =  90⁰, hypotenuse  AC = hypotenuse DF (given) and BC = EF     (given)

 so,  ∆ ABC ≅  ∆ DEF           (R-H-S Condition)

or,  48⁰ = x + 5⁰  and  y + 5 =  12

or,    x  = 43⁰  and  y = 7