CLASS-9
PERIMETER & AREA OF QUADRILATERAL
PERIMETER & AREA OF QUADRILATERALS –
i) Perimeter of a Quadrilateral –
If A, B, C, and D are the lengths of the four sides of a quadrilaterals, then its perimeter = A + B + C + D
ii) Area of a Quadrilateral –
a) When one diagonal and perpendiculars from remaining vertices to the diagonal are given.
Area of quadrilateral-
ABCD = area △ABD + area △BCD
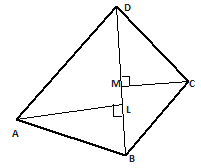
1 1
= ------ X BD X AL + ------ X BD X CM
2 2
1
= ----- BD (AL + CM)
2
1
= ----- X one diagonal X sum of lengths of perpendiculars on it from
2
maintaining vertices.
There are some example is given below for your better understanding -
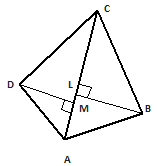
Example.) In a four sided field, the longer diagonal is 110 m. The lengths of the perpendiculars from the opposite vertices upon this diagonal are 18.6 m and 13.4 m respectively. Find the area of the field.
Ans.) Let ABCD be the field and AC be its longer diagonal.
Let, BL ⊥ AC and DM ⊥ AC such that
AC = 110 m, BL = 18.6 m and DM = 13.4 m
1
So, area of the field = [------ X AC X (BL + DM)] m²
2
1
= [------ X 108 X (18.6 + 13.4)] m²
2
= (54 X 32) m² = 1728 m²
So, the area of the field is 1728 m² (Ans.)